Increasing and decreasing functions: Difference between revisions
No edit summary |
No edit summary |
||
| Line 6: | Line 6: | ||
</div> | </div> | ||
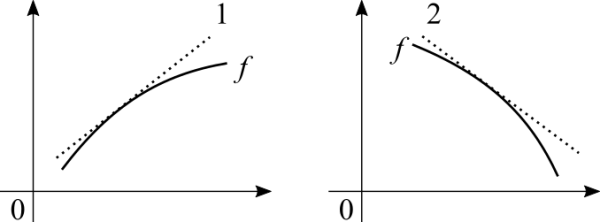
Case 1 the tangent is positive, with a positive rate of change the function is increasing. Case 2 the tangent is negative, with a negative rate of change the function is decreasing. Careful! The above graphs deals with a small closed interval. A function may be always crescent, always decrescent, always constant or a display different behaviours at different intervals. | '''Case 1''' the tangent is positive, with a positive rate of change the function is increasing. '''Case 2''' the tangent is negative, with a negative rate of change the function is decreasing. '''Careful!''' The above graphs deals with a small closed interval. A function may be always crescent, always decrescent, always constant or a display different behaviours at different intervals. | ||
Revision as of 23:31, 19 March 2022
At school we learn to identify crescent and decrescent intervals of functions by inspecting their respective graphs. If the teacher explains the rate of change we can have a better understanding of it. Else we are left with the argument that the graph shows the obvious and no further explanations are given. What we don't learn is that a derivative is a tool that we need to prove that the function is crescent or decrescent in a certain interval.
Case 1 the tangent is positive, with a positive rate of change the function is increasing. Case 2 the tangent is negative, with a negative rate of change the function is decreasing. Careful! The above graphs deals with a small closed interval. A function may be always crescent, always decrescent, always constant or a display different behaviours at different intervals.